The Algebra of warehouse locations: what happens to inventory when you increase the number of distribution centers?

Square Root Law of Inventory Management

Warehouse management can be reduced to mathematics and equations. For many companies, activities tend to orbit the warehouse. Metaphorically, the warehouse, like the sun in the solar system, exerts gravitational forces on your business. Your supply chain, logistics and distribution function in orbits around the warehouse. If you have one large warehouse, the logistics, supply chain (i.e. gravitational forces) are not only easier to manage, they become more cost-effective.

Intuitively, it’s easy to grasp the magnitude of warehouse-scale on your operations. Multiple warehouses tend to increase costs, especially in terms quantity of stock. The main advantage of multiple warehousing — proximity to local customers and some reduced shipping costs — is easily offset by inventory dynamics.

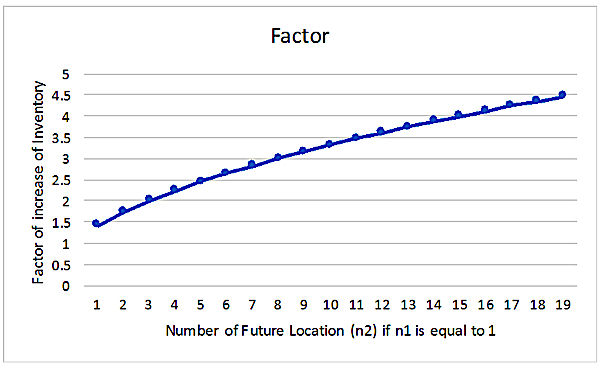
Square Root Law of Inventory Management as a factored chart.

Warehousing by the numbers is non-linear

Simply put, multiple warehouses require significantly more carried inventory, higher rent, extra staffing and added maintenance costs. The offsetting factor is possibly some incremental increases in shipping costs and times.

What may seem less intuitive is the inventory levels increase or decrease in a non-linear way. [See chart.] This is because, if we store products in four locations instead of one, we do not increase our inventory needs by a factor of four. Statistically, the Square Root Law, as first published by D.H. Maister in 1976, takes into account “standard deviation.”

• Would you like one of our warehouse planners to review your current inventory management? Please use our handy form, below.

The equation: Square Root Law of Inventory Management

Putting mathematics to this concept is less intuitive, but adds precision to your planning — whether you are considering consolidation of warehouses or expansion. The Square Root Law of Inventory is also a form of risk pooling, especially in terms of the amount of “safety stock” you have to warehouse. [See Risk Pooling below.]

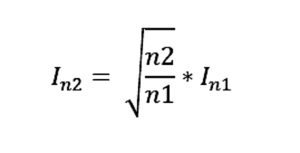
The Square Root of Inventory formula (in our version we substituted “v” for the square root symbol.

The equation of the Square Root Law of Inventory Management is:

X2 = (X1)*v(n2/n1)

The formula may look intimidating, but it becomes clear when you define the variables. The two X-factors are your current inventory (X1) and your future inventory (X2):

• X2 = future inventory
• X1 = existing inventory

The n factors are numbers of locations:

• n1 = number of your existing facilities
• n2 = number of future facilities.

Finally, v is a stand-in for the square root symbol (see inset for proper format of the square root formula. If you are unfamiliar with calculating the square root of fractions, please see inset. When you square root a fraction, you square root both the numerator and the denominator.)

Example: three warehouses consolidated to one

Square roots of fractions are a little more complicated.

Apply your numbers to the equation. Here’s an illustrative example:

• 100,000 –inventoried units are required in stock in your warehouse to service your client’s needs
• Number of warehouses currently: 3
• Number of warehouses you are consolidating to: 1

The resulting equation: X2 = (100,000)*v(1/3)

 

In case you’re not up on your algebra, this results in 57,700 = (100,000) * 0.577

Important note: the square root of 1/3 is 0.577  [See the inserted formula for the square root of 1/3]

What does this mean?

It means you can reduce inventory, potentially to 50% of the current levels by moving to one warehouse from four, lowering costs of space, utilities, staff and — most importantly — carrying inventory. This would typically more than offset any additional shipping costs.

Example 2: Moving from four warehouses to six

On the other hand, assume you’re considering increasing the number of local warehouses (based on the same number of inventoried units) to accommodate new markets.  This formula shows just how costly this plan can be; in addition to extra rent, utilities and staff, you will have to budget for this increase in units:

X2 = (100,000)*v(6/4)

Square root of 6 is 2.449; square root of 4 is 2; solution 2.449/2 [Or, just use an online square root calculator.]

X2= (100,000)*1.225 (square root of 6/4)

In this case, you’ll need 122,500 units and will have to carry the costs of 6 warehouses.

Like any equation, it doesn’t necessarily include all variables. There are other factors, such as local costs (staff, space, utilities), shipping costs, and the costs of inventory management.

What is clear, though, is there are significant advantages to consolidating to fewer, larger warehouses.

When planning distribution, it’s best to take into account the Square Root Law of Inventory Management. Depending on your operation, it can be most cost effective to consolidate.

Example 3: smaller operation

Taking the example of a smaller operation, with a need for 20,000 inventoried units to serve its customers, and currently running four warehouses:

X2 = (20,000)*v(1/4)

X2 = (20,000)*(1/2)  [1/2 is the square root of 1/4]

In this case, the inventory need is reduced to 10,000, or half.

Risk Pooling

Risk pooling is another statistical concept which reduces variability through aggregation. Although there are mitigation factors in diversification, risk pooling analysis tends to support the theme inherent in the Square Root Law of Inventory Management.

How? Having local warehouses means you have smaller orbits (using our previous metaphor) of customers, increasing variability. With increased variability (seasonality, demand, promotions, etc.) comes the requirement of “safety stock.” This can be costly with numerous warehouses.

Consolidating to fewer warehousing, as suggested in the Square Root Law of Inventory Management, means less demand variability and less “safety” stock. Although freight may somewhat increase in this model, most other costs go down, including inventory, staffing, utility,  and rent.

• Would you like one of our warehouse planners to review your current inventory management? Please use our handy form below.